Appendix VI - Lesson 13
Symmetric Harmony
Notes to Appendix VI - Lesson 13
Appendix VI - Lesson 13 is all you ever wanted to know about Symmetric Harmony. The name is enough to scare us away, wouldn't you say? Symmetric harmony is just a complicated term that means your harmony is going to progress in parallel progressions, rather than according to the Cycle of Fourth's. We can move in half steps (minor 2nd), or whole steps (Major 2nd), 3 half steps (minor third's), 4 half steps (Major 3rd's), etc.
The very famous composition known to many, "Holiday For Strings" by David Rose, has a part where he does symmetric harmony in tri-tones, or six half steps. But probably the most familiar use is taking a diminished 7 chord and moving it up or down by a minor third. As the chord is built from minor thirds, each movement is a different inversion of the same chord.
The basic premise is that the first chord in the series is a logical progression from the tune's harmonic structure. The last chord or ending chord of the symmetric phrase must be a logical chord to return to the tune's harmonic structure. We divide the space in between the beginning and ending and put in the same chord form, only moved up or down by the desired step.
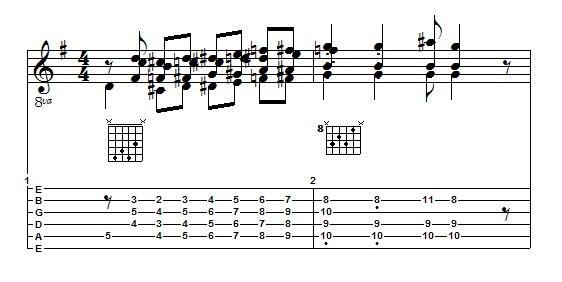
The following example shows symmetric or parallel harmony going from V7 to a I using Dominant 7 chords:

An Example of Symmetric Harmony with a Dominant 7 Chord
A TEF of this example is included in this lesson's TEF's, so either play it or listen to the MIDI playback. You may have heard this in a Blues or Dixieland (traditional Jazz) tune. More commonly used chord forms are, however, diminished 7 forms, and Augmented triads. As an augment triad is built of major thirds, an even number of parallel movements will put us on an inversion of the starting chord.
Mickey says that any chord form we desire can be moved symmetrically, especially in minor thirds as the fourth movement will be the same starting chord, only an octave higher or lower.
Electronics technicians have a term for a module that is fixed and cannot be opened or serviced. It's called a "Black Box". They know what goes into the black box and what is supposed to come out of the black box. If they don't get the desired results, they replace the entire black box. I think of symmetric harmony as a black box. We know the lead-in chord and the exit chord and we divided the space between equally and fill them with parallel chords. Mickey says to think of each of those parallel chords as a tonic. He says one can have up to 12 tonics. To me, that's a bit confusing, so I just think of them as black box harmony. You can use either explanation or invent your own.
Mickey's given us an example by taking an F# dim7 (which can also be called a D# dim7, A dim7, or C dim7) and he moves it four times, each a minor third. Note that each is an inversion of the first. About the most striking example of this particular symmetric harmony with diminished 7's is from the sound track of the silent movies where there is action. To build the suspense, the pianist or organist would play ascending diminished 7 chords. The next time you have the opportunity to watch a silent movie that has action, like a Western, listen for the parallel diminished 7's.
Additional Exercises: This lesson is a little light on work, so after studying it, why not return to Appendix VI - Lesson 12 and write out some more arrangements as we did in that lesson?
Be sure to move your fun up and down symmetrically!!!